
Limits of sums are discussed in detail in the chapter on Sequences and Series however, for now we can assume that the computational techniques we used to compute limits of functions can also be used to calculate limits of sums. First, note that taking the limit of a sum is a little different from taking the limit of a function f ( x ) f ( x ) as x goes to infinity. Some subtleties here are worth discussing. See a graphical demonstration of the construction of a Riemann sum. Figure 5.8 shows the area of the region under the curve f ( x ) = ( x − 1 ) 3 + 4 f ( x ) = ( x − 1 ) 3 + 4 on the interval using a left-endpoint approximation where n = 4. Then, let’s do the same thing in a right-endpoint approximation, using the same sets of intervals, of the same curved region. Let’s explore the idea of increasing n, first in a left-endpoint approximation with four rectangles, then eight rectangles, and finally 32 rectangles. We can demonstrate the improved approximation obtained through smaller intervals with an example. We will have more rectangles, but each rectangle will be thinner, so we will be able to fit the rectangles to the curve more precisely. However, it seems logical that if we increase the number of points in our partition, our estimate of A will improve. Looking at Figure 5.5 and the graphs in Example 5.4, we can see that when we use a small number of intervals, neither the left-endpoint approximation nor the right-endpoint approximation is a particularly accurate estimate of the area under the curve. Sketch left-endpoint and right-endpoint approximations for f ( x ) = 1 x f ( x ) = 1 x on use n = 4. When the left endpoints are used to calculate height, we have a left-endpoint approximation. Then, the sum of the rectangular areas approximates the area between f ( x ) f ( x ) and the x-axis. We find the area of each rectangle by multiplying the height by the width. We determine the height of each rectangle by calculating f ( x i − 1 ) f ( x i − 1 ) for i = 1, 2, 3, 4, 5, 6. We then form six rectangles by drawing vertical lines perpendicular to x i − 1, x i − 1, the left endpoint of each subinterval. In graph (a) we divide the region represented by the interval into six subintervals, each of width 0.5. The graphs in Figure 5.5 represent the curve f ( x ) = x 2 2.

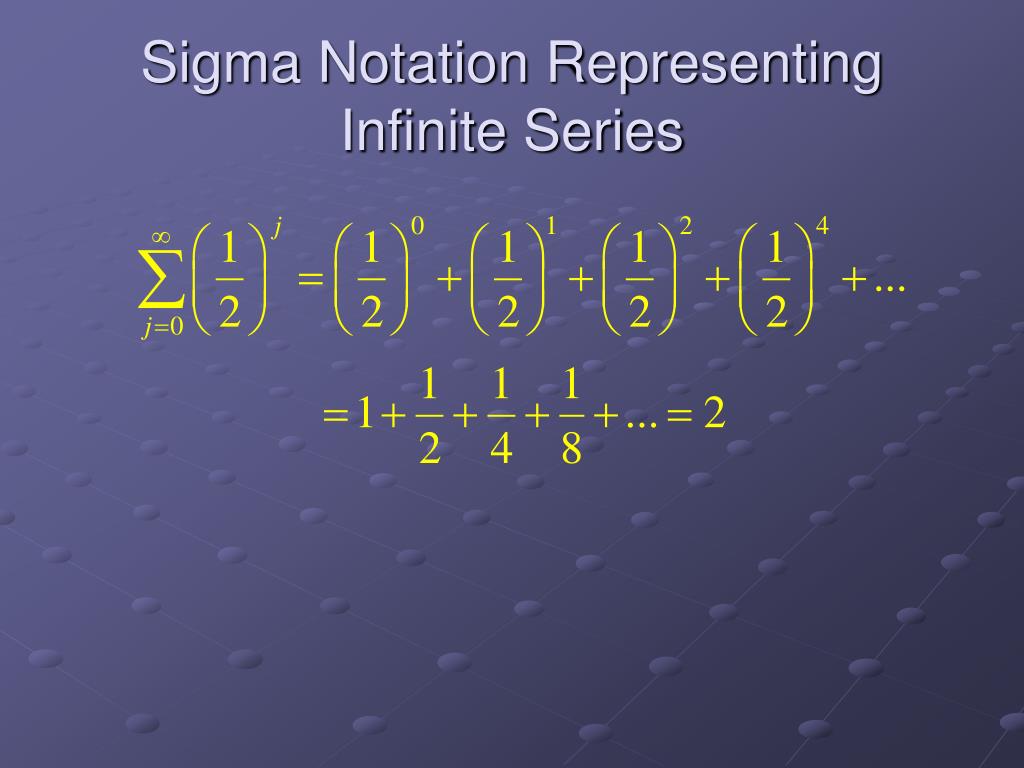
Note that the right-endpoint approximation differs from the left-endpoint approximation in Figure 5.3. For example, if we want to add all the integers from 1 to 20 without sigma notation, we have to writeįigure 5.4 In the right-endpoint approximation of area under a curve, the height of each rectangle is determined by the function value at the right of each subinterval. The Greek capital letter Σ, Σ, sigma, is used to express long sums of values in a compact form. To make it easier to write down these lengthy sums, we look at some new notation here, called sigma notation (also known as summation notation). This process often requires adding up long strings of numbers. Sigma (Summation) NotationĪs mentioned, we will use shapes of known area to approximate the area of an irregular region bounded by curves. Later in the chapter, we relax some of these restrictions and develop techniques that apply in more general cases. We then consider the case when f ( x ) f ( x ) is continuous and nonnegative. Let’s start by introducing some notation to make the calculations easier. Taking a limit allows us to calculate the exact area under the curve. By using smaller and smaller rectangles, we get closer and closer approximations to the area.

Like Archimedes, we first approximate the area under the curve using shapes of known area (namely, rectangles). In this section, we develop techniques to approximate the area between a curve, defined by a function f ( x ), f ( x ), and the x-axis on a closed interval. These areas are then summed to approximate the area of the curved region. In this process, an area bounded by curves is filled with rectangles, triangles, and shapes with exact area formulas. He used a process that has come to be known as the method of exhaustion, which used smaller and smaller shapes, the areas of which could be calculated exactly, to fill an irregular region and thereby obtain closer and closer approximations to the total area.


 0 kommentar(er)
0 kommentar(er)
